Polynomials are fundamental in mathematics, and understanding their roots is critical for various applications in science and engineering. Among the most intriguing aspects of polynomials is the concept of complex roots, especially in the context of nth degree polynomials. In this article, we will delve deep into the nature of complex roots, how they manifest in nth degree polynomials, and the implications of these roots in mathematical theories and real-world applications.
Complex roots arise from the fact that polynomial equations can have solutions that are not real numbers. For an nth degree polynomial, the number of roots is determined by the Fundamental Theorem of Algebra, which states that a polynomial of degree n will have exactly n roots in the complex number system, counting multiplicities. This article aims to explore the types of roots, their properties, and methods to find these complex roots.
By the end of this article, readers will have a comprehensive understanding of complex roots in nth degree polynomials, enhancing their mathematical knowledge and problem-solving skills. Let's embark on this mathematical journey into the world of complex roots!
Table of Contents
- What Are Complex Roots?
- Fundamental Theorem of Algebra
- Properties of Complex Roots
- Finding Complex Roots
- Examples of Complex Roots
- Applications of Complex Roots
- Challenges in Complex Roots
- Conclusion
What Are Complex Roots?
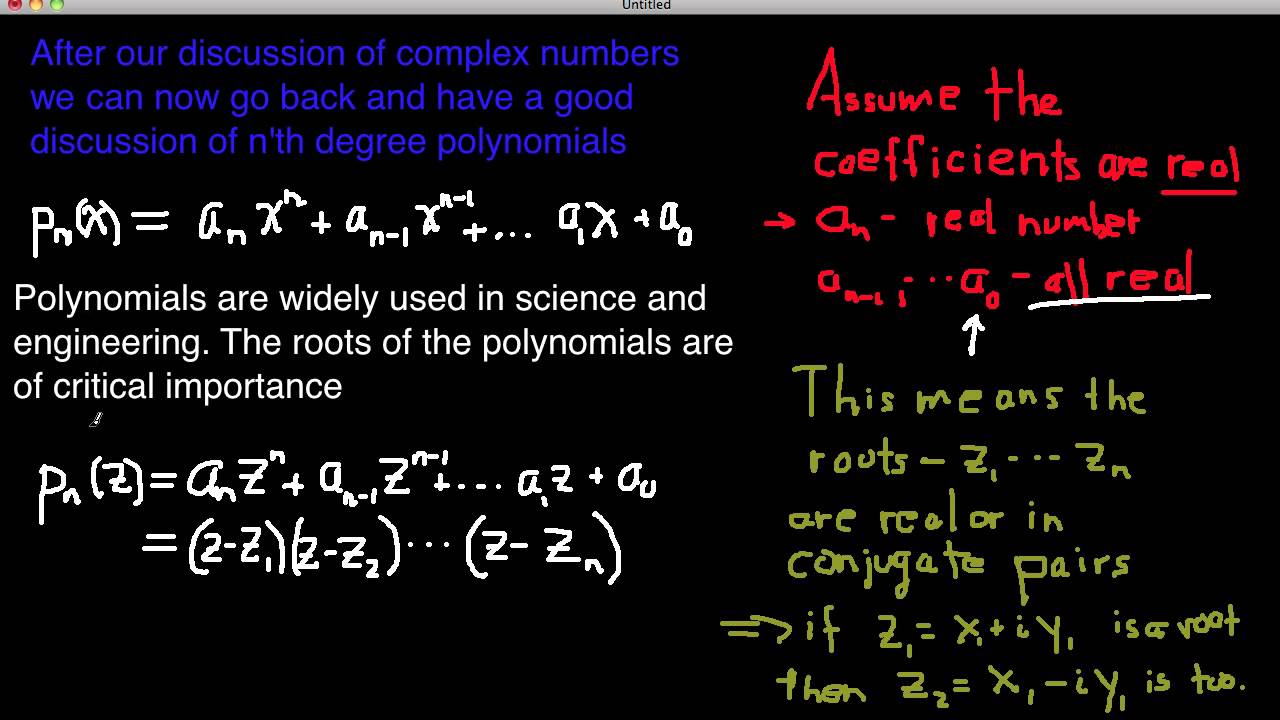
Complex roots are solutions to polynomial equations that involve imaginary numbers. Specifically, a complex number is expressed in the form a + bi, where a and b are real numbers, and i is the imaginary unit, defined as the square root of -1. In the context of polynomials, complex roots can occur in conjugate pairs due to the coefficients of the polynomial being real numbers.
Definition and Examples
For example, consider the polynomial equation:
x² + 1 = 0
Solving this equation yields:
x² = -1
x = ±i
Here, the roots are purely imaginary, demonstrating that not all roots of polynomials are real.
Fundamental Theorem of Algebra
The Fundamental Theorem of Algebra is a pivotal concept in polynomial mathematics, stating that every non-constant polynomial equation of degree n has exactly n complex roots, counting multiplicities. This theorem highlights the completeness of the complex number system in solving polynomial equations.
The theorem can be represented as follows:
- A polynomial of degree n can have:
- n real roots,
- n complex roots,
- or a combination of both.
Implications of the Theorem
The implications of this theorem are profound, suggesting that complex numbers are not merely an abstraction; they are essential for a complete understanding of polynomial equations. This completeness is critical in advanced mathematics, physics, and engineering.
Properties of Complex Roots
Complex roots exhibit several noteworthy properties that distinguish them from real roots. Understanding these properties is crucial for working with polynomials effectively.
- Conjugate Pairs: If a polynomial has real coefficients, complex roots always come in conjugate pairs. For instance, if a + bi is a root, then a - bi is also a root.
- Multiplicity: Roots can have multiplicity greater than one, meaning they can appear more than once as solutions. For example, in (x - 1)² = 0, the root x = 1 has a multiplicity of 2.
- Complex Plane Representation: Complex roots can be represented in the complex plane, where the x-axis represents the real part and the y-axis represents the imaginary part.
Finding Complex Roots
There are several methods for finding complex roots of polynomials, each suitable for different types of equations.
1. Factoring
Factoring is often the simplest method. If a polynomial can be factored into lower degree polynomials, the roots can be easily found. For instance, the polynomial x³ - 3x + 2 can be factored into (x - 1)(x² + x - 2), leading to solutions.
2. Synthetic Division
Synthetic division is a streamlined method for dividing polynomials, which can help in identifying possible rational roots and simplifying the polynomial to find complex roots.
3. Numerical Methods
For polynomials that cannot easily be factored, numerical methods such as Newton's method can be employed to approximate complex roots.
4. The Quadratic Formula
For second-degree polynomials, the quadratic formula x = (-b ± √(b² - 4ac)) / 2a can be used to find roots, including complex ones when the discriminant (b² - 4ac) is negative.
Examples of Complex Roots
Let’s look at a few examples to illustrate how complex roots can be identified in nth degree polynomials.
Example 1: Quadratic Polynomial
Consider the polynomial:
x² + 4 = 0
Using the quadratic formula:
x = ±√(-4) = ±2i
Example 2: Cubic Polynomial
For the polynomial:
x³ + 3x² + 3x + 1 = 0
Factoring gives us (x + 1)³ = 0, meaning x = -1 is a root with multiplicity 3. No complex roots here, but it demonstrates the importance of root multiplicities.
Example 3: Higher Degree Polynomial
For a polynomial such as:
x⁴ + 1 = 0
Factoring gives (x² + 1)(x² - 1) = 0, leading to roots ±i and ±1.
Applications of Complex Roots
Complex roots play a significant role in various fields, including engineering, physics, and computer science.
- Electrical Engineering: Complex numbers are used in electrical engineering to represent impedances in alternating current (AC) circuits.
- Control Theory: Complex roots of the characteristic equation are crucial for stability analysis in control systems.
- Signal Processing: Techniques such as Fourier transforms utilize complex roots to analyze signals in frequency domains.
Challenges in Complex Roots
While understanding complex roots is essential, it also poses challenges, particularly in computation and visualization.
1. Computational Complexity
Finding roots of high-degree polynomials can be computationally intensive, requiring advanced algorithms and numerical methods.
2. Visualization Issues
Visualizing complex roots can be challenging, particularly for those not familiar with the complex plane. Educational tools and software can assist in visualizing these concepts.
Conclusion
In conclusion, the study of complex roots in nth degree polynomials is a fascinating area of mathematics with far-reaching implications. Understanding these roots enhances our ability to solve complex equations and applies to various scientific fields. We encourage readers to explore further, engage with mathematical problems, and share their thoughts on this topic.
If you have enjoyed this article, please consider leaving a comment below, sharing it with others, or exploring our other articles for more insights into the world of mathematics!
Thank you for reading, and we look forward to seeing you again soon!